What is ans. Of ques. 5a and 6 plese see in the attatchment snd repy me

Let us use the following chemical equation: A ---> products.

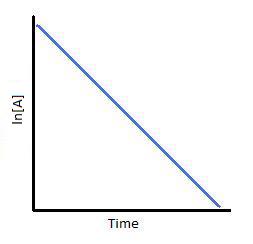
The decrease in the concentration of A over time can be written as: - d[A] / dt = k [A]

Rearrangement yields the following: d[A] / [A] = - k dt

Integrate the equation, which yields: ln [A] = - kt + C

Evaluate the value of C (the constant of integration) by using boundry conditions. Specifically, when t = 0, [A] = [A]_o. [A]_o is the original starting concentration of A.

Substituting into the equation, we obtain: ln [A]_o = - k (0) + C.

Therefore, C = ln [A]_o

We now can write the integrated form for first-order kinetics, as follows:

ln [A] = - kt + ln [A]_o

This last equation can be rearranged into several formats, such as:

ln ([A] / [A]_o) = - kt

[A] / [A]_o = 

[A] = [A]_o 

Question-6:a) If [A]₀ be initial concentration and k be rate constant then using above formula

[A] = [A]_o 

 = 0.8  {For 20% completion, concentration of reactants is [A]₀ – (0.2)[A]₀}

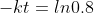
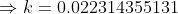 (You can round off the value as per your convenience)

Hence for 75% completion

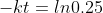
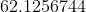
So time taken for 75% completion is 62.126 minutes(approx).

Question-6:b) t_1/2 is called half-life of the radioactive substance which is the time taken for its concentration to become half of its initial concentration.

Using above formula you will get

t_1/2 =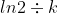 

Also for 99.9% completion time taken is

t =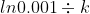 

Hence their ratio is given by (or relation between them is)

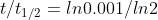
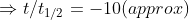
Hope it helps!

regards,

Soumendu