Ask a Doubt
Get your questions answered by the expert for free
Other Related Questions on modern physics


Two particles approach each other from very large distance as shown, . if the least separation between the particles is b, what is the speed of M in center of mass frame when particles are closest? (gravity negligible)
Two particles approach each other from very large distance as shown, . if the least separation between the particles is b, what is the speed of M in center of mass frame when particles are closest? (gravity negligible)
modern physics
0 Answer Available
Last Activity: 2 Years ago

Can the sum of two equal vectors be equal to either vector
Can the sum of two equal vectors be equal to either vector
modern physics
0 Answer Available
Last Activity: 2 Years ago

A nucleus X184 at rest emitt alpha particle With kinetic energy 9.0MeV. The product Nucleus is Y. The values of Binding energy per nucleon of alpha particle & Y differ by 1.5MeV. The values of Binding energy per nucleon of X & Y differ by (n÷230) MeV, then n is
A nucleus X184 at rest emitt alpha particle With kinetic energy 9.0MeV. The product Nucleus is Y. The values of Binding energy per nucleon of alpha particle & Y differ by 1.5MeV. The values of Binding energy per nucleon of X & Y differ by (n÷230) MeV, then n is
modern physics
0 Answer Available
Last Activity: 2 Years ago

SUBJECT: PROBLEM BASED ON LINE DETECTION.
Query: How to apply all the filters for line detection on the following piece of image (provided below)? Explain its Observations to comment on its results.
SUBJECT: PROBLEM BASED ON LINE DETECTION.
Query: How to apply all the filters for line detection on the following piece of image (provided below)? Explain its Observations to comment on its results.
Query: How to apply all the filters for line detection on the following piece of image (provided below)? Explain its Observations to comment on its results.
modern physics
0 Answer Available
Last Activity: 2 Years ago





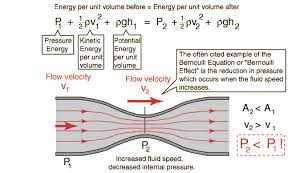
 pressure, ? is fluid density (assumed constant), V is flow velocity, g is the acceleration of gravity and z is the elevation of the fluid particle. The relation applies along any particular
pressure, ? is fluid density (assumed constant), V is flow velocity, g is the acceleration of gravity and z is the elevation of the fluid particle. The relation applies along any particular