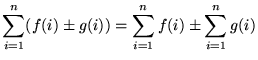Other Related Questions on integral calculus

The area of a square field is 640000cm2.what is its area in hectares
The area of a square field is 640000cm2.what is its area in hectares
integral calculus
1 Answer Available
Last Activity: 2 Years ago

Please solve this integration. I am facing difficulty in it How to solve this. Please solve anyone. Humble request
Please solve this integration. I am facing difficulty in it
How to solve this. Please solve anyone. Humble request
integral calculus
0 Answer Available
Last Activity: 3 Years ago

Using laplace transform, solve d2y/dt2 + dy/dt = t2 +2t given that y=4 and y’=-2 and t=0
Using laplace transform, solve d2y/dt2 + dy/dt = t2 +2t given that y=4 and y’=-2 and t=0
integral calculus
0 Answer Available
Last Activity: 3 Years ago

how to solve this?
this is interger type question .
how to solve this?
this is interger type question .
this is interger type question .
integral calculus
0 Answer Available
Last Activity: 3 Years ago





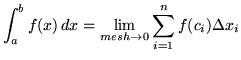 .
.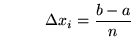
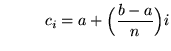
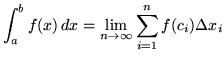 .
.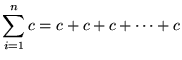 (n times)
(n times) 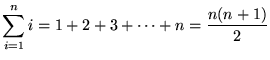
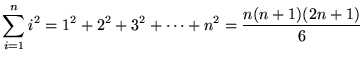
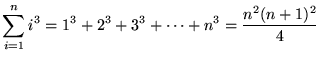
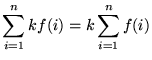 , where
, where