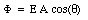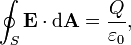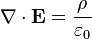Ask a Doubt
Get your questions answered by the expert for free
Enter text here...
Other Related Questions on Discuss with Askiitians Tutors

Please send the full solution as soon as possible 🙏
Discuss with Askiitians Tutors
1 Answer Available
Last Activity: 2 Year ago(s)

I got 80% in jee mains and am from sc category . Will i get a good NIT
Discuss with Askiitians Tutors
1 Answer Available
Last Activity: 2 Year ago(s)

Looking for an integrated JEE+NEET Coaching Classess for 2 years along with an ongoing normal syllabus related classes followed by regular revision tests, please.ThanksGopinath [F/O Class 10th Student-Qatar]
Discuss with Askiitians Tutors
0 Answer Available
Last Activity: 2 Year ago(s)

Ntse coaching for class 9 ncert student, fee and schedule
Discuss with Askiitians Tutors
1 Answer Available
Last Activity: 2 Year ago(s)

Can a class 9 student take admission in the course, 2- what is the duration and mode of full course ie how many student in one class.
Discuss with Askiitians Tutors
0 Answer Available
Last Activity: 2 Year ago(s)





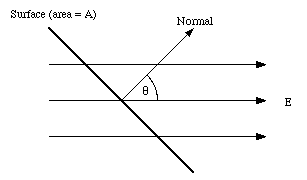
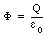 (24.1)
(24.1)