1800-150-456-789
FlagBACK
Flag Differential Calculus> The questions are in the image attached a...
question mark

The questions are in the image attached as a file. Please answer step by step. Thanks a lot.

QuackLaLa , 3 Years ago
Grade 12
anser 1 Answers
Antara

Last Activity: 3 Years ago

51.f(r,n)=n-n^2{nn(1+1/n)(1+1/rn)(1+1/r2n).......}n.        (Taking n common from each bracket)
         = n-n^2 . nn^2 {1+1/n)(1+1/rn).....}n
 Taking log both sides,
ln(f(r,n))=n{ln(1+1/n)+ln(1+1/rn)+ln(1+1/r2n)+......}
 
Taking lim n->inf both sides
RHS=lim(n->inf) {nln(1+1/n)+nln(1+1/rn)+nln(1+1/r2n)+.......}
 
Now lim(n->inf) n *ln(1+1/n)   (inf*0 form of limit)
                        = lim(n->inf)  \frac{ln(1+1/n))}{1/n}
                       =lim(n->inf)    \frac{1/(1+1/n)*(-1/n^2)}{-1/n^2}…............(L hospital rule)
                      = 1
similarly second term=1/r , 3rd term=1/r^2 and so on
so ques 51. ans =e(1+1/r+1/r^2+1/r^3+.......inf)=e3/2  (Putting r=3 and applying sum of inf GP formula)

Provide a better Answer & Earn Cool Goodies

Enter text here...
star
LIVE ONLINE CLASSES

Prepraring for the competition made easy just by live online class.

tv

Full Live Access

material

Study Material

removal

Live Doubts Solving

assignment

Daily Class Assignments


Ask a Doubt

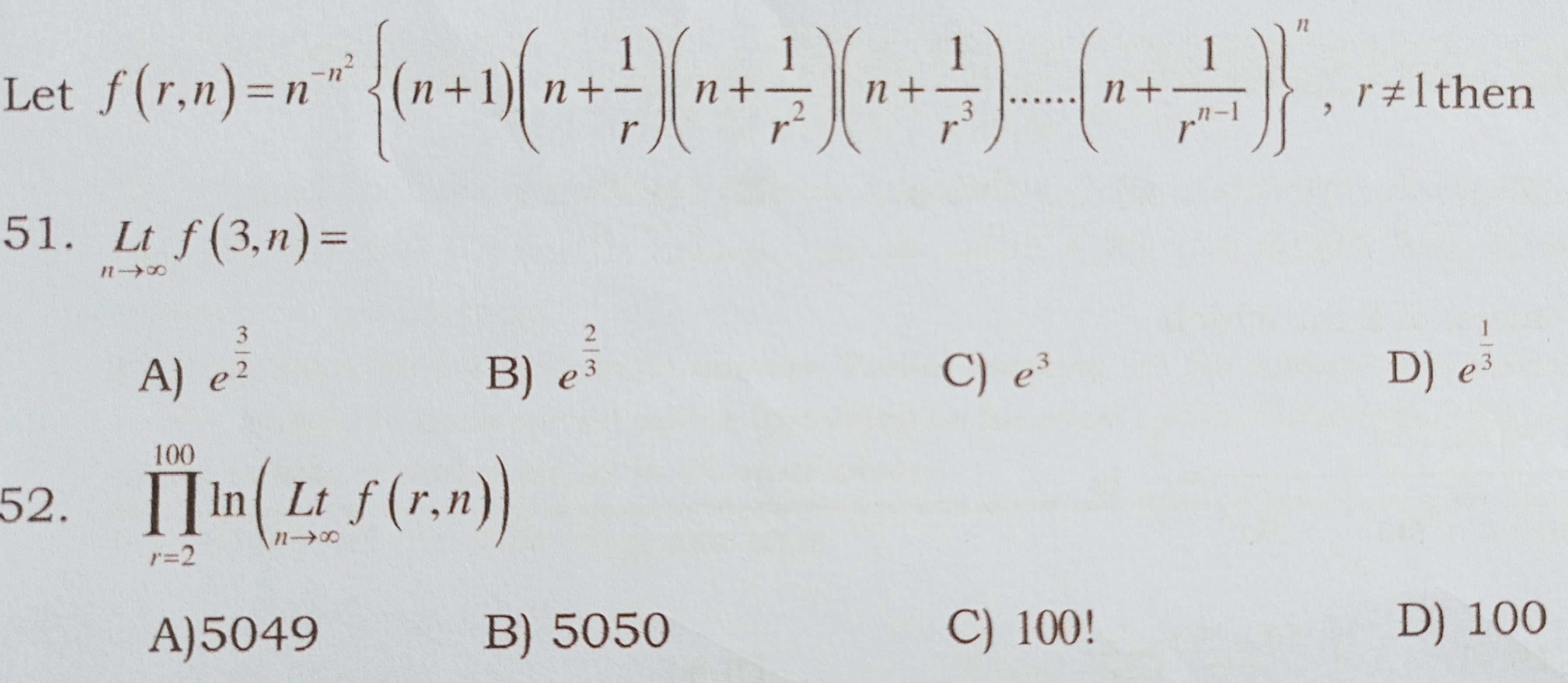
Get your questions answered by the expert for free

Enter text here...

Other Related Questions on differential calculus

question mark
the diameter of a roller is 84 cm and its length is 120 cm. it takes 500 complete revolution tomove once over to level a playground. find the area of the playground in square m?
differential calculus
1 Answer Available

Last Activity: 2 Years ago

question mark
Find dy/DX when y=x^sinx+sin-1√x. Please give me the solution of this problem.
differential calculus
0 Answer Available

Last Activity: 2 Years ago

question mark
If domain of f(x) is [-1, 2], then find the domain of f([x]-x^2+4), where [ ]denotes greatest integer function.
differential calculus
0 Answer Available

Last Activity: 2 Years ago

question mark
Solve the differential equation y’’+2ty’-4y=1, y(0)=y’(0)=0.
differential calculus
0 Answer Available

Last Activity: 2 Years ago

question mark
Example 20. (i) In a AABC, find the maxima and minima of u = sin A sin B sin Cwhere A + B + C = T(ii) In a plane triangle ABC, find the maximum value of cosA cosB cosC.
differential calculus
0 Answer Available

Last Activity: 2 Years ago