Find the value of following limits :Limit x tends 0 ( 7^x -3^x)/(5^x -2^x)
Ankit Prasanna Saket , 7 Years ago
Grade 11
Differential Calculus> Find the value of following limits : Limi...
2 AnswersKailash Jagadeesh
Last Activity: 7 Years ago
Take 3^x common in the numerator and 2^x in the denominator and write the numerator and denominator as separate fractions . Now multiply and divide by x and use the identity: Lim x---> 0 a^x-1/x = ln a
Vikas TU
Last Activity: 7 Years ago
Dear Student,
limx->0 (7x – 3x)/(5x – 2x) = limx->0 (7xln7 – 3xln3)/(5xln5 – 2xln2) = (ln7 – ln3)/(ln5 – ln2)
= ln(7/3)/ln(5/2) = ln(7/3) – ln(5/2).
134.
y'' = 2e-x ------ (1)
y(0) = 1 , y'(0) = 0.
Integrating (1) wrt x, we get y' = -2e-x + c.
Applying the IC y'(0) = 0, we get y'(0) = -2 + c = 0. Therefore c = 2.
Integrating y' = -2e-x + 2 wrt x, we get y = 2e-x + 2x + d.
Applying the IC y(0) = 1, we get y(0) = 2 + d = 1. Therefore d = -1.
Therefore the solution is y = 2e-x + 2x – 1.
Cheers!!
Regards,
Vikas (B. Tech. 4th year
Thapar University)
Thapar University)
Provide a better Answer & Earn Cool Goodies
Enter text here...
LIVE ONLINE CLASSES
Prepraring for the competition made easy just by live online class.
Full Live Access
Study Material
Live Doubts Solving
Daily Class Assignments
Ask a Doubt
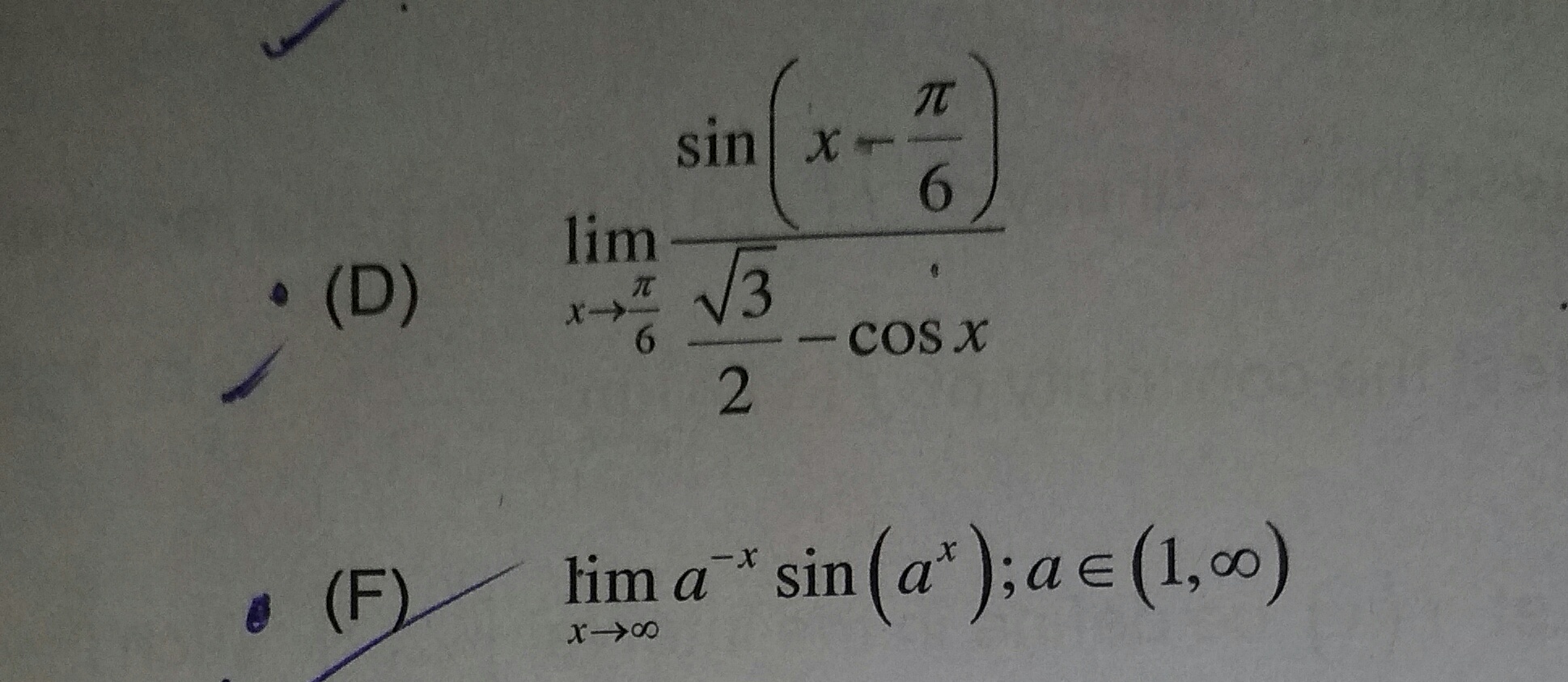
Get your questions answered by the expert for free
Enter text here...
Other Related Questions on differential calculus
the diameter of a roller is 84 cm and its length is 120 cm. it takes 500 complete revolution tomove once over to level a playground. find the area of the playground in square m?
differential calculus
1 Answer Available
Last Activity: 2 Years ago
Find dy/DX when y=x^sinx+sin-1√x. Please give me the solution of this problem.
differential calculus
0 Answer Available
Last Activity: 2 Years ago
If domain of f(x) is [-1, 2], then find the domain of f([x]-x^2+4), where [ ]denotes greatest integer function.
differential calculus
0 Answer Available
Last Activity: 2 Years ago
Solve the differential equation y’’+2ty’-4y=1, y(0)=y’(0)=0.
differential calculus
0 Answer Available
Last Activity: 2 Years ago
Example 20. (i) In a AABC, find the maxima and minima of u = sin A sin B sin Cwhere A + B + C = T(ii) In a plane triangle ABC, find the maximum value of cosA cosB cosC.
differential calculus
0 Answer Available
Last Activity: 2 Years ago